go to WEEK 3 Problem 1 2 3 4 5 Demo

MSE 5090: Case Studies in Material Selection

Week 3 - Material Selection Process

 Weighted Property Index Methods - Problem 3

The race to build "monster" optical telescopes is getting really exciting. A number of groups are currently working toward the construction of "optical giants," with two to three times the light-gathering power of the largest optical telescope in the Western world, the 5-rn Hale device situated atop Mount Palomar, Calif.

(a) It is true that the trend toward larger optical telescopes is one of the most exciting fields in applied physics today.

Thermomechanical loading and distortion considerations require that the mirror materials possess the following characteristics:
·Almost zero coefficient of thermal expansion a, in order to minimize dimensional change due to thermal growth. From structural and systems considerations, the thermal expansivities of the mirror as well as the support structure (including the mirror mounting fixtures) must be nearly equal

Microstructural homogeneity and isotropy of a, in order to minimize spatial gradients
in a (i.e., da/dx ® 0, where x is the spatial dimension).

· Relative insensitivity of a to temperature change in the region of interest (i.e., da/dT ® 0).
· High thermal diffusivity a, in order to dissipate the absorbed incident energy.
· Low scattering of light, or else high compatibility with antireflection coatings.
· High specific elastic stiffness (E/p). to enable fabrication of a mechanically stable mirror.
· Rapid and easy preparation for final polishing and coating.
· High microyield strength s_y¢ , so that all strains in service are totally elastic.
· Using the simple theory of flexure of a plate under uniform loading (arising from self-weight). The magnitude of the maximum sag or deflection A for a circular mirror, simply supported continuously around its periphery (Fig. Q54.2), is given by [1]

where W is the total self-weight of the mirror. v is the Poisson’s ratio of the mirror material, R is the outside radius of the mirror, and t is its wall thickness. Taking the average value of the Poisson’s ratio of most nonmetallic mirror materials to be 0.23,*

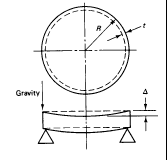Figure Q54.2 Deflection of a circular solid mirror supported continuously around its edges.

we have an approximate expression for D given by 

where C is a constant of magnitude 0.24. It is thus clear from equation (Q54.l) that to minimize the central deflection. E must be as high as possible; to minimize self-weight, for a given mirror stiffness (W/D), it can be shown that the material property index (p³/E)^0.5 must be as low as possible.

(b) The values of the relevant properties of some of the more promising materials for these mirrors and comments on them are presented in Tables Q54.2 and Q54.3.

(c) Three approaches toward developing large optical surfaces are currently in use. The thin-meniscus approach uses a mirror that has a thickness of only about 2—5 cm. The optical surface is kept at a very accurate level (~0.5 mm) by a set of computer-controlled supports located on the reverse of the mirror that exerts alternating tensile and compressive force at the membrane of material. The magnitude of this applied force is approximately equal and opposite to the distortional gravity forces. The thin meniscus technology has been demonstrated in the 3.5-m New Technology Telescope for the European Southern Observatory.

The second approach is the segmented mirror concept. This involves the formation of the precision optical surface as an intricate array of hexagonal pieces that are aligned to a precision of about 100 nm through use of computer-controlled supports. This technology is used in the Keck telescope.

The third approach is the honeycomb design in which the precision optical surface is cast by spinning the mold, resulting in a paraboloid shape. Following the cooling of the material and the freezing of the curve, the surface is given a final polishing. The major advantage of this approach is that it completely dispenses with the need for grinding out the basic curve from a slab of the optical material. This approach is to be used in the United States’ National New Technology Telescope.

*The value of v for fused silica is 0.167; for ULE 7971 it is 0.l76; for Zerodur 543561 it is 0.24; for CG4 it is 0.121; and for borosilicate glass it is 0.21.

TABLE Q54.2 SOME PROPERTIES OF SELECTED CANDIDATE MATERIALS FOR PRECISION OPTICS COMPONENTS

^aThermal diffusivity = k/(pc_p), where k is the conductivity, p is the density, and c_p is the specific heat.
^bUltralow expansion glass: 92.5% SiO₂—7.5% TiO₂; registered trademark of Corning Glass Works, Coming, N.Y.
^c2MgO *2Al₂O₃*5SiO_2.
^dVolume coeflicient of thermal expansion = (a along a-axis + a along c-axis)/3.
^e2MgO* 2Al₂O₃*4SiO₂* GeO₂.

1. E. J. Hearn, Mechanics of Materials. vol. 2. Oxford: Pergamon Press Ltd., p. 632. 1985.


Last update 9-12-98